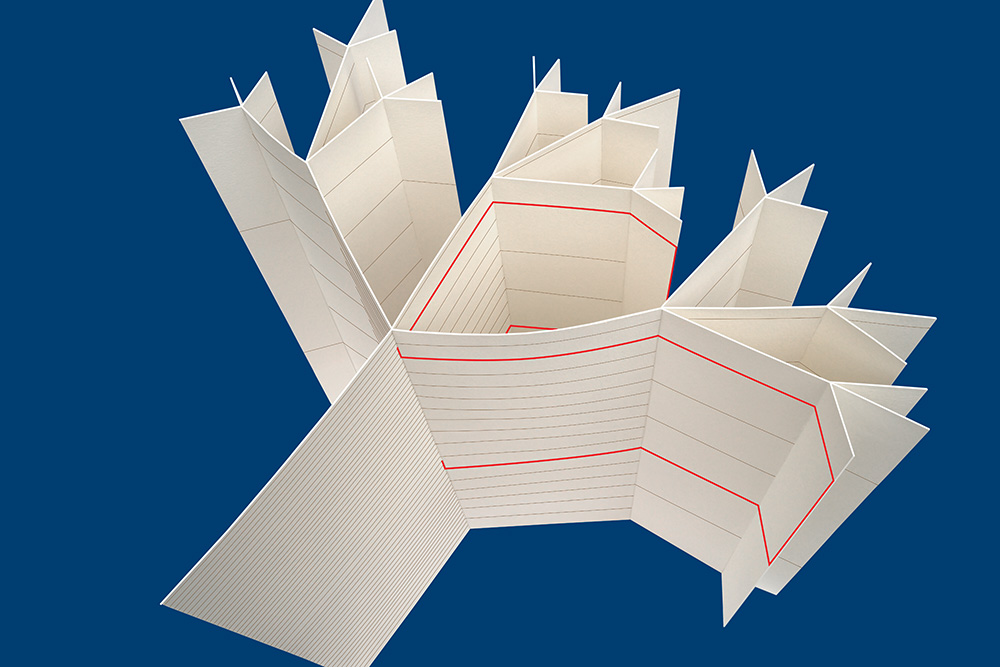
I just finished making a small contribution to this image (hopefully to appear on the cover of the Proceedings of the National Academy of Sciences) built by Tammy Cantarella and Aaron Abrams.
The structure illustrates some of Aaron’s research on Dehn functions. Aaron’s work is way deeper than this example, but the example is still pretty neat. It shows a space where a curve can enclose exponentially much area compared to its length (as opposed to curves in the plane, which can only enclose area proportional to the square of their length). The rectangles on the complex above are each considered to have the same area. At each branch point, the lines split off along the three leaves of the tree in cyclically repeating order, so the number of lines on each leaf is one-third of the number on the “parent” leaf. This means that a curve which goes far out from the central spine can enclose a lot of rectangles near the spine with a very short length.
Tammy made this picture by actually building the tree from paper and carefully photographing it. Then she edited the image in Photoshop and added the horizontal and vertical lines. Aaron provided the math, and I provided a little design advice and mostly translated between the two of them.
Kudos to Tammy and Aaron– this looks awesome!